

Accurate Powder Measurement

|

|
Mechanical Powder Scales (RCBS and CH)
Every muzzleloader shooter or reloader needs to measure powder as accurately as desired or at least possible under the given
circumstances. You can measure powder by volume or by weight. In the old days, some people put a lead ball on their palm and
poured gunpowder over it until it was completely covered. Today, this early form of volumetric measurement appears a little
rustic to us, and no serious sport shooter would want to measure his powder in such a manner. I am not even too enthusiastic
about the volumetric powder measures you can buy because accurate volumetric measurement of solid materials relies on a uniform
bulk density. The latter, however, may be subject to variations (caused by storage conditions, vibrations, etc.) when working
with powdered or granular material.
Being a chemist by profession, I definitely prefer gravimetric measurement (volumetry is for liquids and gases). Of course, you
have more work to do, and you need a decent powder scale. If you do it right, however, you will be rewarded with a more reproducible
powder weight which translates into more uniform shooting results. While black powder is rather forgiving, smokeless powder is less
so (smaller dosage, much higher peak pressure). Therefore, particularly reloaders using smokeless powder may consider measuring
their powder with a scale or at least use a scale to cross-check their powder charges on a regular basis. PLEASE, do NOT use a
kitchen scale or letter scale for this purpose, use a dedicated powder scale.
I didn’t pay much attention to my old CH powder scale for a long time because most manufacturers claim a sensitivity of 0.1 grain,
and even an error of ±1 grain (1 gr = 64.79891 milligrams) is not a disaster when shooting with black powder handguns. I became
more aware of weighing errors, however, when I purchased a Smith & Wesson 686 revolver and started hand-loading ammunition.*
*I load most of my .357 Magnum cartridges with a 180 gr TC lead bullet and 7.0 gr smokeless powder (Vihtavuori N340). This is a comfortable-to-shoot target load with a moderate recoil (slightly stronger than an average .38 Special round).
The following measurements were performed with two mechanical scales (RCBS 10-10 and CH). I do NOT use electronic powder scales since I found them rather inaccurate and unreliable (a REALLY good electronic scale is much more expensive than any powder scale). At the beginning of the test series, I set each scale at zero weight and then adjusted the beam pointer to match with the zero mark by turning the levelling screw in the base of the scale. Afterwards, I cross-checked the weights displayed by the scales against a set of calibration weights from a chemical lab. Before starting the (single) measurements, I had verified the accuracy of the calibration weights with a freshly calibrated analytical scale (sensitivity: 0.1 mg ≈ 0.0015 gr). This is what I found:
|
Calibration Weight [gr] |
Error RCBS [gr (%)] |
Error CH [gr (%)] |
| 00.00 | ±0.00 (±0.00) | ±0.00 (±0.00) |
| 05.00 | -0.10 (-2.00) | -0.05 (-1.00) |
| 10.00 | -0.10 (-1.00) | +0.05 (+0.50) |
| 15.00 | -0.20 (-1.33) | ±0.00 (±0.00) |
| 20.00 | -0.25 (-1.25) | +0.05 (+0.25) |
| 25.00 | -0.25 (-1.00) | -0.05 (-0.20) |
| 30.00 | -0.20 (-0.67) | +0.15 (+0.50) |
| 35.00 | -0.20 (-0.57) | +0.05 (+0.14) |
| 40.00 | -0.25 (-0.63) | +0.10 (+0.25) |
| 45.00 | -0.20 (-0.44) | -0.05 (-0.11) |
| 50.00 | -0.30 (-0.60) | ±0.00 (±0.00) |
The results show that the weighing error is not constant but varies with the chosen weight range. The CH scale performed better than the
RCBS 10-10. The errors are not dramatic but they reveal a common misconception about the accuracy of powder scales. A sensitivity of
0.1 gr, which is often stated in data sheets, does NOT mean the weighing error is smaller than 0.1 gr from the beginning.
The weighing error comprises two components which are superimposed upon each other, the systematic error and the random error. The
systematic error is a repetitive offset of the weight measured by the scale from the actual (true) weight, e. g., a calibration
weight.* In contrast, the random error causes the weight displayed by the scale to scatter around an average value when we repeat
a measurement several times. With a single cross check against a calibration weight, we can only determine the momentary sum of
both error components, the total error. To separate both components from each other, we need a greater number of cross checks
against the same calibration weight. The systematic error is usually defined as the difference between the arithmetic mean of the
displayed values and the calibration weight. The random error is described by the standard deviation of the displayed values. This
method is widely used but it relies on a certain type of symmetric error distribution (Gauss curve), and the results get distorted
when outliers exist. These have to be identified and removed if necessary. Alternatively, one can define the systematic error as
the difference between the median of all data points and the calibration weight. Using this method, the random error can be
characterized, for example, by the interquartile range of all data points. This alternative method is much less sensitive to
outliers ("robust statistics"). Therefore, I decided to use it here.
The terms "accuracy" and "precision" are often confused with each other. Accuracy refers to the systematic error of a scale (or
any other instrument) while precision refers to the random error (high accuracy = small systematic error, high precision = small
random error). By calibration, we improve the accuracy of an instrument but not its precision. Precision is a measure for the
reproducibility of measurement values and for the ability of a scale to indicate small weight changes (sensitivity). For example,
a scale with a sensitivity of 0.1 gr may very well display an average weight of 20.5 gr while the true weight is 20.0 gr
(systematic error: 0.5 gr), but it will show a measurable movement of the beam pointer as soon as you increase the true weight to
20.1 gr or decrease it to 19.9 gr.
The sensitivity of a powder scale is largely determined by its geometry, for example, by the vertical distance between the bearing
of the beam and its center of gravity. However, a low sensitivity is not necessarily the result of a bad design but may have other
reasons, for example, static friction in the bearings of the beam or the suspension of the weighing pan. Dirt and wear increase the
bearing friction, so the bearings should be kept clean and not be exposed to excessive load or shocks. Poor accuracy or precision may
also be caused by a careless reading technique (parallax!). Make sure your eye is always on a level with the zero mark on the dial
plate when checking the position of the beam pointer.
The results shown above demonstrate that it is NOT sufficient to set a scale with unknown properties at the desired weight and
then trickle powder into the weighing pan until the beam pointer comes to rest at the zero position. If you want to measure
powder accurately, you first have to make yourself familiar with the properties of the scale and then calibrate it against a
suitable reference weight every time before measuring a batch of powder charges (do not move the scale around after calibration).
In this way, you directly compare the powder weight with the reference weight(s) and minimize the systematic error of the scale.
This is the procedure:
|
1. Put the scale on a flat, firm surface. Make sure the scale is not exposed to any air draft. |
|
2. Set the scale at the desired weight. |
|
3. Put the corresponding reference weight(s) into the weighing pan. |
|
4. Turn the levelling screw until the beam pointer lines up with the zero graduation on the dial plate. |
|
5. Remove the reference weight(s) and start weighing the powder charges. |
To identify systematic and random error of a scale with unknown properties, you need a greater number of measurements (the
greater the number the better) and perform a statistical analysis.
I did a very simple exploratory data analysis. After calibrating the scale against a 7.00-grain reference weight, I measured
a batch of 16 powder charges of 7.0 grains each. Afterwards, I checked the charges with an analytical scale (the same one as
used above). A sample of only 16 data points is probably not ideal for reliable statistics, but it may at least give you an
impression of the performance of a typical mechanical powder scale. I started with the RCBS 10-10 scale. Next, I repeated the
procedure with my old CH scale (CH 1). Both studies were done under lab conditions, i. e., as precisely as possible. In addition, I
checked an older batch of powder charges measured with the (calibrated) CH scale under normal workshop conditions. This batch
(CH 2) had been prepared before (!) I thought about doing a statistical study, so the test results are unbiased.
Here are the five-number summaries of the errors with respect to the target value (measured in grains). If you are not familiar with
the statistical terms, you can find their explanation in Wikipedia. The numbers
become more graspable when each column of the table is visualized in the form of a box plot:
|
|
Here is a short explanation for those not familiar with box plots: Each plot consists of a rectangular box with two vertical
"antennas" attached to it. On a vertical scale, the end of the lower antenna marks the smallest value, the end of the upper
antenna the greatest value of the whole data set. The horizontal line inside the box represents the median of all values. The
lower side of the box is the median of those values smaller than the median of the whole data set (1st quartile), the upper
edge of the box is the median of those values greater than the median of the whole data set (3rd quartile). Thus, we find the
smallest 25% of all values along the lower antenna, the greatest 25% of all values along the upper antenna, and the remaining
50% of all values inside the box. Each vertical length represents a data range. The height of the box, for example, indicates
the interquartile range (IQR).
What does this study tell us? First of all, the calibration error, approximately represented by the offset of the median from
the true weight, is negligible in all three cases. It is slightly different from zero because no calibration is 100% accurate
due to reading errors and the limited sensitivity of the scale. Even the greatest total (systematic + random) error observed
in this study is smaller than the specified scale sensitivity of 0.1 gr.
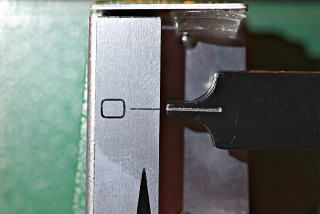
Obviously, the old CH scale is more sensitive than the (more expensive) RCBS scale when both are used with equal care (CH 1). I
found this confirmed when observing the movement of the beam pointer after putting a 0.1 gr weight into the weighing pan of each
scale. The following photos (same magnification factor) demonstrate the pointer movements:
 |
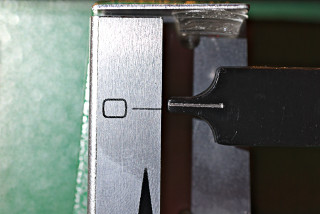 |
| RCBS 10-10 Scale, 0.0 gr | RCBS 10-10 Scale, +0.1 gr |
 |
 |
| CH Scale, 0.0 gr | CH Scale, +0.1 gr |
Under workshop conditions (CH 2), the total scale error is, of course, greater than in the lab but still tolerable (< 0.1 gr),
provided the scale has been calibrated as explained above. After learning these results, I decided to use the RCBS scale for
measuring black powder and the CH scale for measuring smokeless powder in the future.
Sets of calibrated reference weights for powder scales are available as reloading accessories. If you have access to a reliable
scale, you can make your own set at almost no cost. I purchased a small coil of stainless-steel wire in a hardware store. After
degreasing the wire with acetone, I determined the weight of the wire per unit length. Then I cut pieces of wire which were slightly
heavier than the respective target weight. Using an analytical scale, a wire cutter, and a file, I tuned each piece of wire to the
exact target value. In this way, I made the following reference weights (see photo): 0.5 gr, 1.0 gr, 2.0 gr (2x), 5.0 gr, 7.0 gr,
10.0 gr, and 20.0 gr (2x). I further made a tiny 0.1 gr weight (not shown) to check the sensitivity of scales.
|
|
|
Home-Made Calibration Weights (from left to right: 0.5 gr, 1.0 gr, 2.0 gr, 5.0 gr, 7.0 gr, 10.0 gr) |
Volumetric Measurement:
I was curious to see how reproducible powder measurement by volume actually is. Since I didn't have anything better available,
I used a 9 mm Luger cartridge case as a powder measure. I filled it with Vihtavuori N 340 powder, removed excessive powder by
moving the edge of a ruler across the opening of the case, and weighed the full case with an analytical scale. I performed
this procedure with the same case 16 times, carefully avoiding any shocks or vibrations which might have changed the bulk
density of the powder. The five-number summary of the data set (net weight) thus collected is shown below (together with box
plot):
|
|
To have a direct comparison with the gravimetric measurements shown above, I calculated the interquartile range (IQR) of each data set. The IQR (= 3rd quartile minus 1st quartile), which includes 50% of the data points, is a measure for the scattering of data points (similar to the standard deviation). The following bar graph shows the IQRs of the four test series:
|
|
The IQR of the volumetric measurements is about four times greater than the IQR of the measurents done with the CH scale under
practical conditions (CH 2). This is ok for black powder charges but smokeless powder is a different story. The greatest deviation
from the median I observed was about 0.138 gr. With regard to a 7-grain charge, this would be an error of approx. 2%. No big deal
for everyday use but maybe a little too much for precision shooting. It depends on the individual shooter's requirements.
Afterword:
Nobody would want to weigh 500 powder charges per week (we have still other things to do). If you want to make small batches of
your own, high-quality ammunition, however, weighing the powder should be considered. Of course, this is more work than measuring
powder with small cups. View it as a meditative exercise. The way (weight) is the goal.

